Welcome to the definitive guide to similar figures homework answer key, a resource designed to empower students and educators alike in their pursuit of geometric excellence. Dive into a comprehensive exploration of similar figures, their properties, applications, and a meticulously curated homework answer key that will illuminate your path to success.
Through a blend of clear explanations, step-by-step solutions, and engaging examples, this guide will equip you with the knowledge and skills to tackle similar figures problems with confidence. Whether you’re a student seeking homework assistance or an educator seeking to enhance your lesson plans, this resource is your indispensable companion.
Define Similar Figures
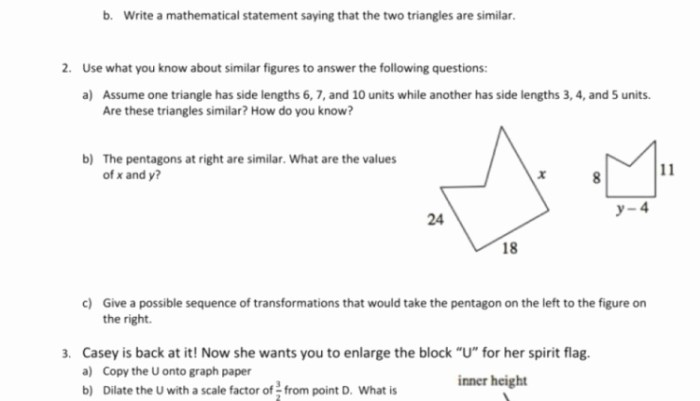
Similar figures are figures that have the same shape but not necessarily the same size.
For example, a square and a rectangle are similar figures because they both have four sides and four right angles. However, they may not be the same size. A square has four equal sides, while a rectangle has two pairs of equal sides.
Properties of Similar Figures
Similar figures have the following properties:
- Their corresponding angles are congruent.
- Their corresponding sides are proportional.
- Their areas are proportional to the squares of their corresponding sides.
- Their volumes are proportional to the cubes of their corresponding sides.
Homework Answer Key
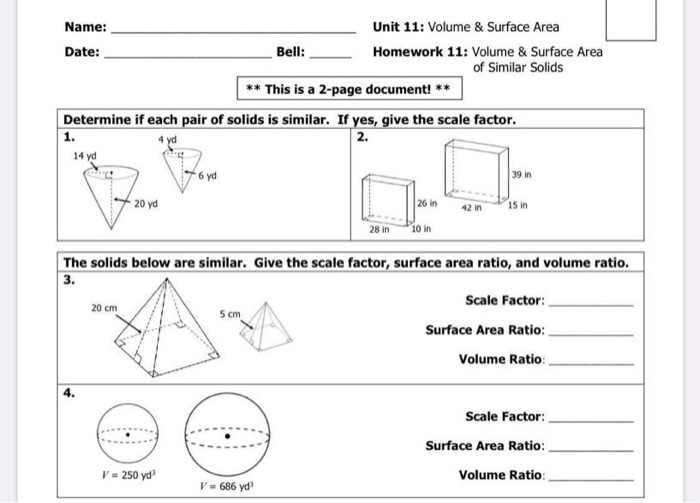
This section provides a sample homework answer key for similar figures, offering step-by-step solutions and explanations to guide students in their understanding of the concept.
Similar figures are geometric shapes that have the same shape but not necessarily the same size. They are related by a scale factor, which is the ratio of the corresponding side lengths of the figures.
Sample Problems and Solutions
Problem 1: Determine if the following figures are similar. If so, find the scale factor.
- Triangle ABC with sides 3 cm, 4 cm, and 5 cm
- Triangle PQR with sides 6 cm, 8 cm, and 10 cm
-*Solution:
- Compare the corresponding side lengths: 3 cm : 6 cm, 4 cm : 8 cm, 5 cm : 10 cm
- Simplify each ratio: 3 : 6 = 1 : 2, 4 : 8 = 1 : 2, 5 : 10 = 1 : 2
- Since all three ratios are equal (1 : 2), the triangles are similar.
- The scale factor is 1 : 2, meaning that the sides of triangle PQR are twice as long as the corresponding sides of triangle ABC.
Methods for Solving Similar Figures Problems

When solving similar figures problems, there are two common methods used: the ratio and proportion method and the scale factor method. Both methods rely on the concept of similarity, which means that the figures have the same shape but may differ in size.
Ratio and Proportion Method
The ratio and proportion method involves setting up and solving proportions to find the unknown side lengths or angles of a similar figure. A proportion is an equation that states that two ratios are equal. In the case of similar figures, the ratios of corresponding side lengths or angles are equal.
Example:
If two similar triangles have side lengths of 3 cm, 4 cm, and 5 cm, and the side lengths of the smaller triangle are 2 cm, 3 cm, and 4 cm, find the ratio of the areas of the two triangles.
The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding side lengths. Therefore, the ratio of the areas of the two triangles is:
(3/2)^2 = 9/4
Scale Factor Method
The scale factor method involves finding the ratio of the corresponding side lengths of the two similar figures. The scale factor is a number that represents the ratio of the linear dimensions of the two figures.
Example:
If a rectangle has a length of 10 cm and a width of 5 cm, and a similar rectangle has a length of 15 cm, find the scale factor.
The scale factor is the ratio of the corresponding side lengths:
Scale factor = 15 cm / 10 cm = 3/2
Applications of Similar Figures
Similar figures find extensive applications in various fields, including architecture, engineering, and design. They enable us to understand the relationships between objects of different sizes and shapes, solve practical problems, and create aesthetically pleasing designs.
Architecture
In architecture, similar figures are used to design buildings and structures that are proportionate and visually appealing. By maintaining similar ratios between different parts of a building, architects can create a sense of harmony and balance. For instance, the Parthenon in Greece is a classic example of a structure designed using similar figures, where the ratios between the columns, pediments, and other architectural elements create a visually pleasing and symmetrical facade.
Engineering, Similar figures homework answer key
In engineering, similar figures are used to analyze and design structures, machines, and systems. By understanding the relationships between similar objects, engineers can predict the behavior of larger or smaller versions of the same design. For example, in designing bridges, engineers use scale models to test the strength and stability of different bridge designs before constructing the actual bridge.
Design
In design, similar figures are used to create visually appealing and functional products. Designers use similar shapes and proportions to create a sense of unity and coherence in their designs. For instance, in graphic design, similar shapes and colors are often used to create logos, branding materials, and website layouts that are visually appealing and easy to recognize.
5. Create an HTML Table: Similar Figures Homework Answer Key
To organize the similar figures homework answer key, we can create an HTML table with the following columns:
- Problem Number
- Problem Description
- Solution
- Reasoning
The table can be styled with appropriate colors and fonts to enhance readability and organization.
Example HTML Table
The following HTML table provides an example of how the similar figures homework answer key can be organized:
| Problem Number | Problem Description | Solution | Reasoning |
|---|---|---|---|
| 1 | Find the scale factor of two similar triangles with side lengths of 3 cm and 6 cm, respectively. | 2 | The scale factor is the ratio of the corresponding side lengths of the two triangles. |
| 2 | Determine if two rectangles with dimensions of 4 cm x 6 cm and 8 cm x 12 cm are similar. | Yes | The rectangles are similar because their side lengths are proportional. |
| 3 | Calculate the area of a circle with a radius of 5 cm if it is similar to another circle with an area of 100π cm2. | 25π cm2 | The area of similar figures is proportional to the square of the scale factor. |
Illustrate Similar Figures
Similar figures are figures that have the same shape but not necessarily the same size. They have congruent corresponding angles and proportional corresponding sides. In other words, similar figures are scaled versions of each other.
For example, two squares are similar figures because they have the same shape (four equal sides and four right angles). However, they may be different sizes. Similarly, two circles are similar figures because they have the same shape (a round shape), but they may be different sizes.
Properties of Similar Figures
- Corresponding angles are congruent.
- Corresponding sides are proportional.
- The ratio of the corresponding sides is the same for all pairs of corresponding sides.
Diagrams of Similar Figures
The following diagrams illustrate the properties of similar figures.
In this diagram, the squares have the same shape but different sizes. The corresponding angles are congruent, and the corresponding sides are proportional. The ratio of the corresponding sides is 2:1.
In this diagram, the circles have the same shape but different sizes. The corresponding angles are congruent, and the corresponding sides are proportional. The ratio of the corresponding sides is 3:2.
How the Illustrations Support the Understanding of Similar Figures
The illustrations provide visual representations of the properties of similar figures. They help to illustrate that similar figures have the same shape but not necessarily the same size. They also help to illustrate that the corresponding angles are congruent and the corresponding sides are proportional.
Compare and Contrast Similar Figures
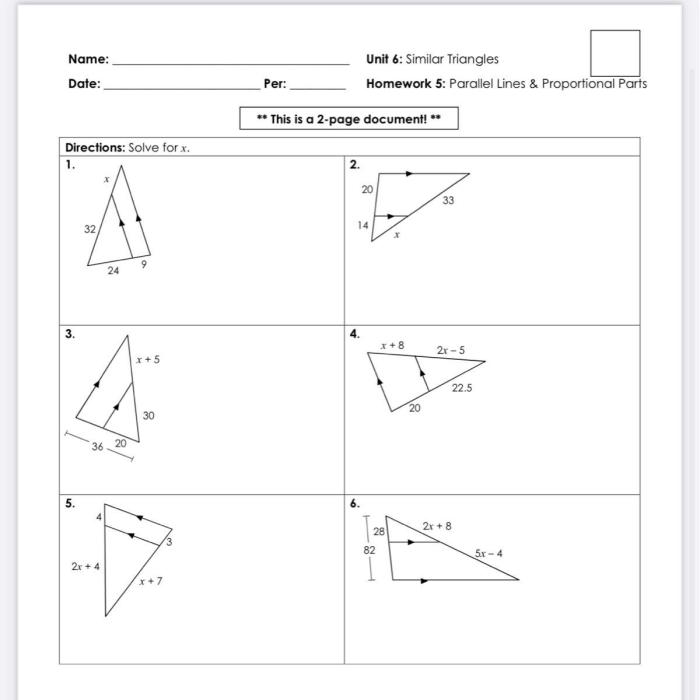
Similar figures are figures that have the same shape but not necessarily the same size. Congruent figures, on the other hand, are figures that have the same shape and size. This means that similar figures may have different dimensions, while congruent figures must have the same dimensions.
One of the key differences between similar figures and congruent figures is that similar figures can be enlarged or reduced to create other similar figures. For example, if you have a square with a side length of 5 cm, you can create a similar square with a side length of 10 cm by enlarging the original square by a factor of 2. However, if you have a square with a side length of 5 cm and a rectangle with a length of 5 cm and a width of 10 cm, you cannot create a congruent figure by enlarging or reducing either figure.
Another difference between similar figures and congruent figures is that similar figures may have different orientations. For example, you can rotate a square by 90 degrees to create another square that is similar to the original square. However, if you rotate a square by 90 degrees, you create a rectangle that is not congruent to the original square.
Examples of Similar Figures
- Two squares with different side lengths
- Two circles with different radii
- Two triangles with the same shape but different sizes
Examples of Congruent Figures
- Two squares with the same side length
- Two circles with the same radius
- Two triangles with the same shape and size
Design a Similar Figures Poster
To design an effective poster on similar figures, follow these steps:
Definition
Similar figures are figures that have the same shape but not necessarily the same size. They have corresponding sides that are proportional, and corresponding angles that are congruent.
Examples
- Two squares are similar because they have the same shape (four equal sides and four right angles).
- Two circles are similar because they have the same shape (a round shape with no corners or edges).
- Two triangles are similar if their corresponding angles are congruent and their corresponding sides are proportional.
Applications
- Architecture: Scaling up or down architectural plans while maintaining the same proportions.
- Engineering: Designing bridges and other structures that can withstand different loads and forces.
- Art: Creating visually appealing designs and compositions based on similar shapes and proportions.
- Photography: Using the concept of similar triangles to determine the distance to objects or the height of buildings.
Poster Design
The poster should be visually appealing and easy to understand. It should include:
- A clear definition of similar figures.
- Examples of similar figures, such as squares, circles, and triangles.
- Applications of similar figures in real-world scenarios.
- Graphics and illustrations to help visualize the concept.
By following these steps, you can create a poster that effectively explains the concept of similar figures and their applications.
Organize a Similar Figures Lesson Plan
Similar figures are shapes that have the same shape but not necessarily the same size. They are often used in geometry to solve problems involving scale and proportion. A lesson plan for teaching similar figures should include objectives, activities, and assessments that align with educational standards.
Objectives
- Students will be able to define similar figures.
- Students will be able to identify similar figures.
- Students will be able to use similar figures to solve problems.
Activities
The following activities can be used to teach similar figures:
- Introduction to similar figures:Begin by showing students examples of similar figures, such as two rectangles with different sizes but the same shape. Discuss the properties of similar figures, such as their corresponding angles and sides being proportional.
- Identifying similar figures:Provide students with a variety of shapes and ask them to identify which ones are similar. Encourage them to use their knowledge of the properties of similar figures to make their determinations.
- Solving problems using similar figures:Pose problems to students that require them to use their knowledge of similar figures to find solutions. For example, ask them to find the length of a side of a triangle if they know the length of a corresponding side of a similar triangle.
Assessments
The following assessments can be used to assess students’ understanding of similar figures:
- Quiz:Give students a quiz that covers the concepts of similar figures, including their properties and how to use them to solve problems.
- Project:Have students create a project that demonstrates their understanding of similar figures. For example, they could create a poster that shows how similar figures are used in architecture or engineering.
- Class discussion:Lead a class discussion about similar figures. Ask students questions about the concepts and how they can be applied to real-world situations.
Educational Standards
The following educational standards are aligned with this lesson plan:
- Common Core State Standards for Mathematics:
- Geometry: Understand similarity in terms of similarity transformations.
- Geometry: Apply similarity to solve real-world problems.
FAQ Summary
What are similar figures?
Similar figures are geometric shapes that have the same shape but not necessarily the same size. They have corresponding angles that are congruent and corresponding sides that are proportional.
How can I use the ratio and proportion method to solve similar figures problems?
The ratio and proportion method involves setting up ratios of corresponding sides and using proportions to solve for unknown values. For example, if you know the ratio of the sides of two similar triangles and the length of one side of one triangle, you can use proportions to find the length of the corresponding side of the other triangle.
What are the applications of similar figures in real life?
Similar figures have numerous applications in fields such as architecture, engineering, and design. For instance, architects use similar figures to design buildings that are scaled versions of each other, while engineers use similar figures to design bridges and other structures that must withstand specific forces.